Consideration of air bubble dynamics in low-pressure hydraulic systems
Pipe resonances and other vibration phenomena in hydraulic systems are strongly influenced by the speed of sound in the pressure medium. In order to be able to reproduce the original system behaviour as accurately as possible during CAE-aided system design or simulation-supported troubleshooting, the speed of sound in the individual components must be known exactly. Among other things, this quantity is influenced by the air bubbles in the pressure medium, whose dynamic effects have not been taken into account so far. In a research project sponsored by the Federal Ministry of Economic Affairs and Energy of Germany (BMWi), FLUIDON is developing a calculation method in cooperation with the renowned Institute for Fluid Power Drives and Systems (ifas) at the RWTH Aachen University in order to be able to cover these effects in the hydraulic simulation with DSHplus.
Project background and intention
The speed of sound in a hydraulic component is determined by the following influencing variables:
-
Stiffness of the pressure fluid (pressure and temperature dependent)
-
Stiffness of the component's housing (e.g. pipe wall)
-
Stiffness of undissolved air components (especially at low pressures!)
Due to the low stiffness of the gas bubbles, the speed of sound of the liquid-air mixture can sometimes be more than one order of magnitude lower than that of the pure liquid. Up to now, the influence of the gas bubbles on the mixture stiffness has only been considered by a quasi-static calculation approach in practically all commercially available software solutions. In this approach, it is assumed that the individual air bubbles can be combined into a single gas volume which behaves like an ideal-elastic spring.
As experiments have shown, this assumption is only valid for vibrations with very low frequency. For larger dynamics, it must be taken into account that the gas bubbles must additionally displace the surrounding liquid particles and overcome their internal friction when their size changes, so that the effective stiffness of the gas bubbles becomes frequency-dependent.
Since the size of the bubbles (and thus the quantity of liquid particles involved) is also important for the dynamic effects, the number and diameter of the individual bubbles are represented in the form of a statistical frequency distribution. For efficient calculation, the different bubble sizes are summarized in size classes ("clusters").
The first important intermediate result of the research project is an equation for calculating the time- and frequency-dependent sonic velocity of the liquid-air mixture, whereby the bubble dynamics are modelled by the so-called Rayleigh-Plesset equation (RPG).
Frequency response of the sound velocity of a liquid-gas mixture with only one bubble size
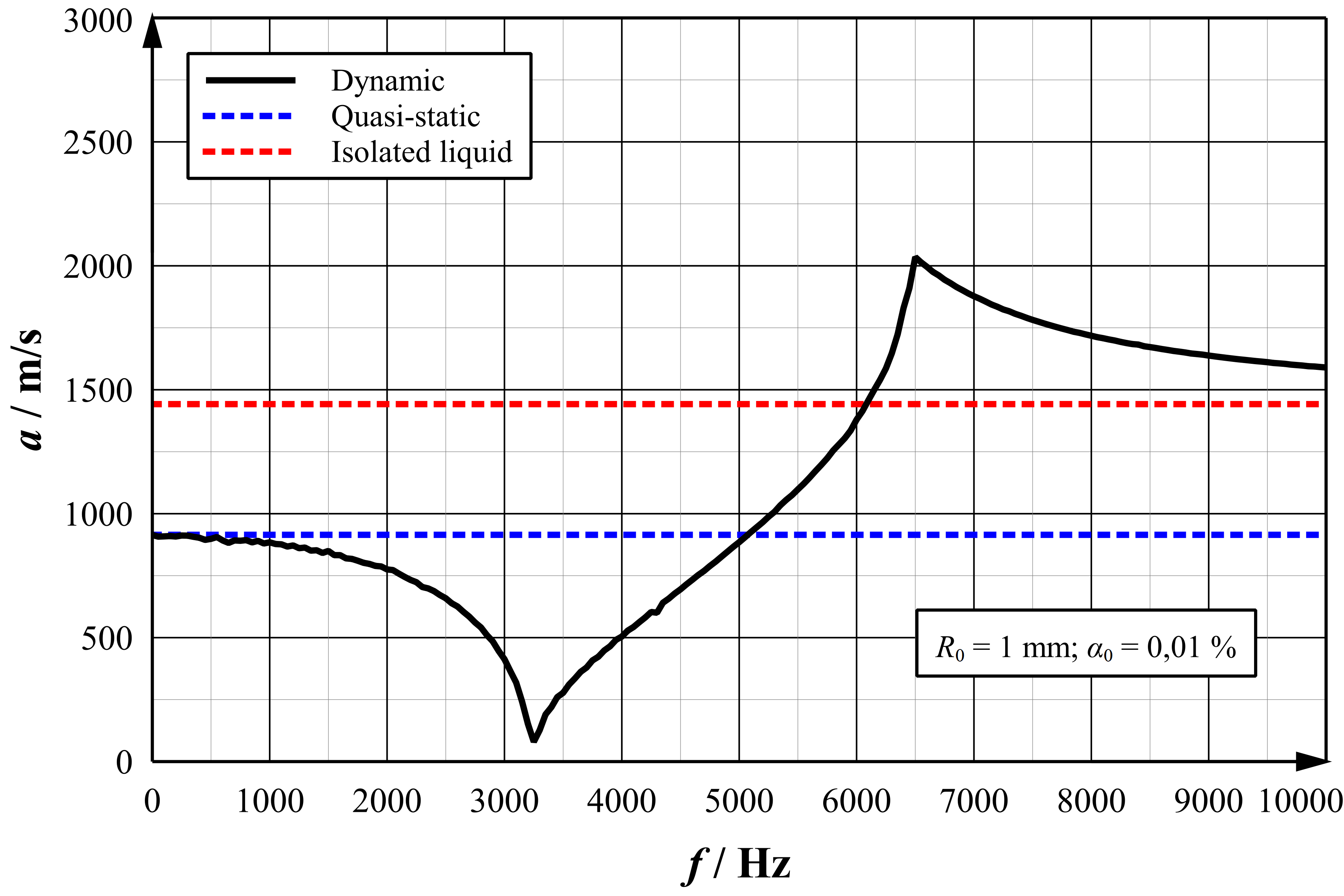
The frequency response of the speed of sound calculated according to this method is shown in the adjacent figure for an exemplary selected mixture with one bubble size.
For very low frequencies, the speed of sound of the liquid-gas-mixture approaches the quasi-static solution, neglecting the bubble dynamics (state of the art). The pronounced minimum (here: 3200 Hz) of the speed of sound corresponds to the resonance frequency of the bubble size; the effective stiffness of the gas bubble is particularly low due to the inertial forces at this frequency. Since the inertial forces modeled by the RPG lead to a progressive stiffening of the bubbles at high frequencies, only the compressibility of the liquid remains in this range, so that the mixture sound velocity tends to converge against that of the isolated liquid.
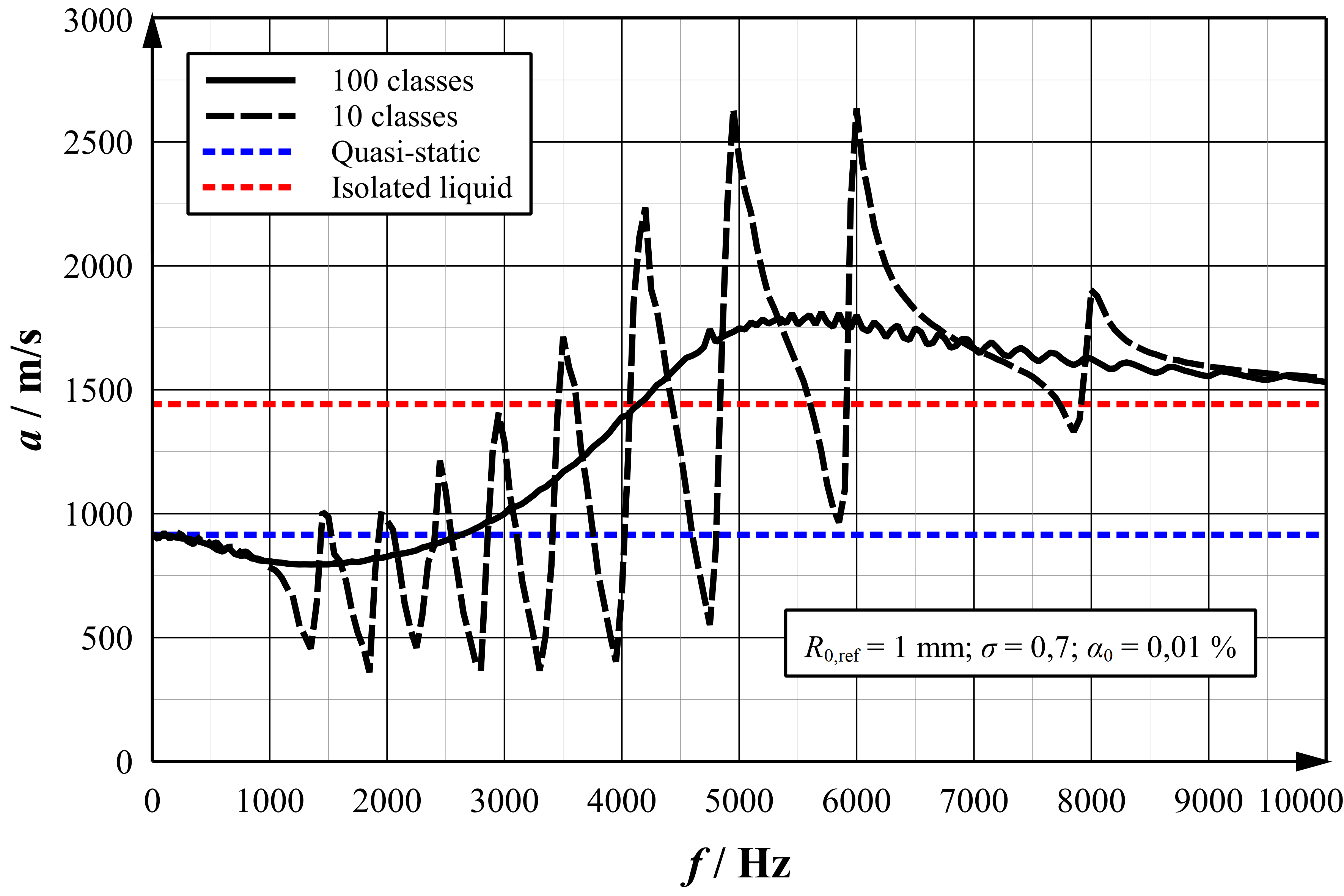
Frequency response of the sound velocity of a liquid-gas mixture with different bubble sizes
A corresponding calculation for a mixture with a bubble distribution is shown in the adjacent figure.
To illustrate the influence of the cluster size, the frequency responses were calculated for 10 and 100 classes. The pronounced minima in the frequency response for 10 classes correspond to the resonance frequencies of the individual bubble sizes. The maxima and minima for 100 classes are much less sharply delineated, since the frequency (and thus the volume fraction) of the individual bubble sizes is correspondingly lower for a larger number of classes.
The frequency response for 100 classes is practically indistinguishable from the continuous solution (not shown here).
The developed numerical scheme is currently being integrated into the tried and tested method of characteristics (MOC) scheme for the mathematical analysis of hydraulic systems.